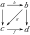Traced monoidal category

In category theory, a traced monoidal category is a category with some extra structure which gives a reasonable notion of feedback.

A traced symmetric monoidal category is a symmetric monoidal category C together with a family of functions

${\displaystyle \mathrm {Tr} _{X,Y}^{U}:\mathbf {C} (X\otimes U,Y\otimes U)\to \mathbf {C} (X,Y)}$

called a trace, satisfying the following conditions:

• naturality in ${\displaystyle X}$: for every ${\displaystyle f:X\otimes U\to Y\otimes U}$ and ${\displaystyle g:X'\to X}$,

${\displaystyle \mathrm {Tr} _{X',Y}^{U}(f\circ (g\otimes \mathrm {id} _{U}))=\mathrm {Tr} _{X,Y}^{U}(f)\circ g}$

• naturality in ${\displaystyle Y}$: for every ${\displaystyle f:X\otimes U\to Y\otimes U}$ and ${\displaystyle g:Y\to Y'}$,

${\displaystyle \mathrm {Tr} _{X,Y'}^{U}((g\otimes \mathrm {id} _{U})\circ f)=g\circ \mathrm {Tr} _{X,Y}^{U}(f)}$

• dinaturality in ${\displaystyle U}$: for every ${\displaystyle f:X\otimes U\to Y\otimes U'}$ and ${\displaystyle g:U'\to U}$

${\displaystyle \mathrm {Tr} _{X,Y}^{U}((\mathrm {id} _{Y}\otimes g)\circ f)=\mathrm {Tr} _{X,Y}^{U'}(f\circ (\mathrm {id} _{X}\otimes g))}$

• vanishing I: for every ${\displaystyle f:X\otimes I\to Y\otimes I}$, (with ${\displaystyle \rho _{X}\colon X\otimes I\cong X}$ being the right unitor),

${\displaystyle \mathrm {Tr} _{X,Y}^{I}(f)=\rho _{Y}\circ f\circ \rho _{X}^{-1}}$

• vanishing II: for every ${\displaystyle f:X\otimes U\otimes V\to Y\otimes U\otimes V}$

${\displaystyle \mathrm {Tr} _{X,Y}^{U}(\mathrm {Tr} _{X\otimes U,Y\otimes U}^{V}(f))=\mathrm {Tr} _{X,Y}^{U\otimes V}(f)}$

• superposing: for every ${\displaystyle f:X\otimes U\to Y\otimes U}$ and ${\displaystyle g:W\to Z}$,

${\displaystyle g\otimes \mathrm {Tr} _{X,Y}^{U}(f)=\mathrm {Tr} _{W\otimes X,Z\otimes Y}^{U}(g\otimes f)}$

• yanking:

${\displaystyle \mathrm {Tr} _{X,X}^{X}(\gamma _{X,X})=\mathrm {id} _{X}}$

(where ${\displaystyle \gamma }$ is the symmetry of the monoidal category).

Properties

• Every compact closed category admits a trace.
• Given a traced monoidal category C, the Int construction generates the free (in some bicategorical sense) compact closure Int(C) of C.

References

• Joyal, André; Street, Ross; Verity, Dominic (1996). "Traced monoidal categories". Mathematical Proceedings of the Cambridge Philosophical Society. 119 (3): 447–468. Bibcode:1996MPCPS.119..447J. doi:10.1017/S0305004100074338. S2CID 50511333.

• v
• t
• e

Category theory

Key concepts

Key concepts Category Abelian Additive Concrete Pre-abelian Preadditive Bicategory Adjoint functors CCC Commutative diagram End Exponential Functor Kan extension Morphism Natural transformation Universal property Universal constructions Limits Terminal objects Products Equalizers Kernels Pullbacks Inverse limit Colimits Initial objects Coproducts Coequalizers Cokernels and quotients Pushout Direct limit Algebraic categories Sets Relations Magmas Groups Abelian groups Rings (Fields) Modules (Vector spaces) Constructions on categories Free category Functor category Kleisli category Opposite category Quotient category Product category Comma category Subcategory

Higher category theory

Key concepts Categorification Enriched category Higher-dimensional algebra Homotopy hypothesis Model category Simplex category String diagram Topos n-categories Weak n-categories Bicategory (pseudofunctor) Tricategory Tetracategory Kan complex ∞-groupoid ∞-topos Strict n-categories 2-category (2-functor) 3-category Categorified concepts 2-group 2-ring En-ring (Traced)(Symmetric) monoidal category n-group n-monoid

• Category
• Outline
• Glossary

This category theory-related article is a stub. You can help Wikipedia by expanding it.

• v
• t
• e